Like this article? We recommend
Like this article? We recommend
Like this article? We recommend
LSD string sort
The first string-sorting method that we consider is known as least-significant-digit first (LSD) string sort. Consider the following motivating application: Suppose that a highway engineer sets up a device that records the license plate numbers of all vehicles using a busy highway for a given period of time and wants to know the number of different vehicles that used the highway. As you know from Section 2.1, one easy way to solve this problem is to sort the numbers, then make a pass through to count the different values, as in Dedup (page <?>). License plates are a mixture of numbers and letters, so it is natural to represent them as strings. In the simplest situation (such as the California license plate examples at right) the strings all have the same number of characters. This situation is often found in sort applications—for example, telephone numbers, bank account numbers, and IP addresses are typically fixed-length strings.
)
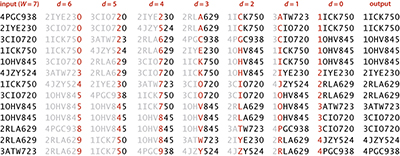
Figure 5.6 Typical candidate for LSD string sort
Sorting such strings can be done with key-indexed counting, as shown in Algorithm 5.1 (LSD) and the example below it on the facing page. If the strings are each of length W, we sort the strings W times with key-indexed counting, using each of the positions as the key, proceeding from right to left. It is not easy, at first, to be convinced that the method produces a sorted array—in fact, it does not work at all unless the key-indexed count implementation is stable. Keep this fact in mind and refer to the example when studying this proof of correctness:
Proposition B. LSD string sort stably sorts fixed-length strings.
Proof: This fact depends crucially on the key-indexed counting implementation being stable, as indicated in Proposition A. After sorting keys on their i trailing characters (in a stable manner), we know that any two keys appear in proper order in the array (considering just those characters) either because the first of their i trailing characters is different, in which case the sort on that character puts them in order, or because the first of their ith trailing characters is the same, in which case they are in order because of stability (and by induction, for i-1).
Algorithm 5.1: LSD string sort
public class LSD
{
public static void sort(String[] a, int W)
{ // Sort a[] on leading W characters.
int N = a.length;
int R = 256;
String[] aux = new String[N];
for (int d = W-1; d >= 0; d--)
{ // Sort by key-indexed counting on dth char.
int[] count = new int[R+1]; // Compute frequency counts.
for (int i = 0; i < N; i++)
count[a[i].charAt(d) + 1]++;
for (int r = 0; r < R; r++) // Transform counts to indices.
count[r+1] += count[r];
for (int i = 0; i < N; i++) // Distribute.
aux[count[a[i].charAt(d)]++] = a[i];
for (int i = 0; i < N; i++) // Copy back.
a[i] = aux[i];
}
}
}
To sort an array a[] of strings that each have exactly W characters, we do W key-indexed counting sorts: one for each character position, proceeding from right to left.
)
Another way to state the proof is to think about the future: if the characters that have not been examined for a pair of keys are identical, any difference between the keys is restricted to the characters already examined, so the keys have been properly ordered and will remain so because of stability. If, on the other hand, the characters that have not been examined are different, the characters already examined do not matter, and a later pass will correctly order the pair based on the more significant differences.
LSD radix sorting is the method used by the old punched-card-sorting machines that were developed at the beginning of the 20th century and thus predated the use of computers in commercial data processing by several decades. Such machines had the capability of distributing a deck of punched cards among 10 bins, according to the pattern of holes punched in the selected columns. If a deck of cards had numbers punched in a particular set of columns, an operator could sort the cards by running them through the machine on the rightmost digit, then picking up and stacking the output decks in order, then running them through the machine on the next-to-rightmost digit, and so forth, until getting to the first digit. The physical stacking of the cards is a stable process, which is mimicked by key-indexed counting sort. Not only was this version of LSD radix sorting important in commercial applications up through the 1970s, but it was also used by many cautious programmers (and students!), who would have to keep their programs on punched cards (one line per card) and would punch sequence numbers in the final few columns of a program deck so as to be able to put the deck back in order mechanically if it were accidentally dropped. This method is also a neat way to sort a deck of playing cards: deal them into thirteen piles (one for each value), pick up the piles in order, then deal into four piles (one for each suit). The (stable) dealing process keeps the cards in order within each suit, so picking up the piles in suit order yields a sorted deck.
In many string-sorting applications (even license plates, for some states), the keys are not all be the same length. It is possible to adapt LSD string sort to work for such applications, but we leave this task for exercises because we will next consider two other methods that are specifically designed for variable-length keys.
From a theoretical standpoint, LSD string sort is significant because it is a linear-time sort for typical applications. No matter how large the value of N, it makes W passes through the data. Specifically:
Proposition B (continued). LSD string sort uses ~7WN+3WR array accesses and extra space proportional to N+R to sort N items whose keys are W-character strings taken from an R-character alphabet.
Proof: The method is W passes of key-indexed counting, except that the aux[] array is initialized just once. The total is immediate from the code and Proposition A.
For typical applications, R is far smaller than N, so Proposition B implies that the total running time is proportional to WN. An input array of N strings that each have W characters has a total of WN characters, so the running time of LSD string sort is linear in the size of the input.